-
Viewpoint on 'IONS'
Viewpoint on 'Scientific Literacy'
- Proudly sponsored by
-

-
Traffic Lights for Chemotherapy
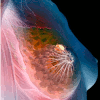
Many cancer patients do not respond to chemotherapy and have to endure harmful side effects even when the treatment itself does not prove effective. A new technique could, as of the very first day, give the green or red light to continuing with the treatment.
-
Outclassing Superheroes

Superheroes, watch out! A new rival, such as you have never come up against before, is in town. Progress is the name! And the exclusivity of your superpowers may well be at stake.
-
Quantum Circuits: Fast and Cool

In the 20th century information technologies based on microelectronics changed the world. In the 21st century nanophotonics looks very likely to become a key factor in the building of quantum information technologies.
Volume 10 Story 3 - 16/8/2010

What happens to a photon when it enters a material? Does it gain momentum, as proposed by Minkowski? Or does it lose momentum, as argued by Abraham? Many great scientists have dwelt on the so-called Abraham-Minkowski dilemma over the past hundred years. Now, Stephen Barrett from the University of Strathclyde, Glasgow, UK, may have solved the puzzle in a brilliant, yet simple way: Abraham and Minkowski are both right!
The origin of the Abraham-Minkowski dilemma can be traced back to the beginnings of the XX century: in 1908 Minkowski suggested that a photon gains momentum when entering a medium; only a year later, in 1909, Abraham contended that, on the contrary, it actually loses momentum. Astonishingly, there are very good reasons to give credit to both viewpoints. Let us have a closer look at some of the most intuitive arguments in favor of each of the two theories.
If a photon traveling in vacuum enters a material, a slab of glass for example, its speed decreases by a factor, known as the refractive index. It follows rather logically that the photon’s momentum, which is proportional to its speed, should also decrease in the glass. There, one might justifiably think, Abraham must be right!
However, a photon is a quantum entity with a wavelength and a momentum given by the Planck’s constant divided by its wavelength. In the glass the wavelength decreases by a factor equal to the refractive index and, therefore, its momentum must increase. We would now seem to be justified in thinking that Minkowski must be right, after all.
Puzzling! Both arguments come across as sound and logical – yet they would also seem to lead to antithetical conclusions. Indeed, for over one hundred years, generations of great scientists racked their brains over this dilemma, unable to reach any sort of definitive resolution.
Since physics is an experimental science, it may be argued that it is for the experiments to decide on the issue. And there is, indeed, very good experimental evidence in favor of the theories – actually, in favor of both theories! Where, then, is the catch, if indeed there is one?
Some experimental evidence in favor of Minkowski comes from the cooling of atoms and from the optical stretcher. The latter, for example, is a device where a cell kept between two counter-propagating laser beams stretches along the axes of the beams. This appears to be consistent with the Minkowski momentum, since the stretching results from the decrease of the momentum when light crosses the boundary between the water and the cell. On the opposite side of the field, sympathetic to Abraham, we find a recent experiment that shows the recoiling of an optical fiber on the exit of a light pulse.
Barnett may well have found the answer. Surprisingly, both arguments are reasonable and sound because they are both right – but they refer to two different momenta, namely the kinetic momentum, where Abraham’s theory is concerned, and the canonical momentum, where Minkowski’s theory is concerned.
The kinetic momentum of a body is simply the product of its mass multiplied by its speed. The kinetic momentum of a photon when it enters a medium decreases in accordance with Abraham’s prediction, but such change is compensated by the recoiling of the medium, so that what is conserved is the total kinetic momentum of the photon and the medium.
The canonical momentum is a little subtler to grasp. It can be intimately related to the quantum nature of the photon. It appears in the interactions between single photons and single atoms, such as atom cooling. The canonical momentum of a photon increases when it interacts with an atom, and, as predicted by Minkowski once again what is conserved is the total canonical momentum of the photon and the atom.
"If light propagates in free space," explains Barnett, "there is no difference between the kinetic and the canonical momentum. However, such difference matters when light propagates inside a medium." This may go some way towards explaining why the fact that Abraham’s and Minkowski’s theories refer to two different momenta, went unnoticed before
Looking ahead, Barnett asks: "Might there be phenomena where both momenta show up at the same time?" Once the idea that both momenta are correct is accepted, it would make sense to plan experiments where both are present together. The focus on trying to prove/disprove Abraham or Minkowski may have prevented scientists from exploring such possibilities in the past – the good news is that this may be about to change soon.

Abraham vs. Minkowski 1-1
Does a photon gain or does it lose momentum when it enters a glass slab? Both may be the simple, yet ingenious answer to this centenary dilemma.
How does the momentum of a photon change when it interacts with matter? Does it increase or decrease? Both viewpoint are actually correct, depending on which momentum is being considered.
The origin of the Abraham-Minkowski dilemma can be traced back to the beginnings of the XX century: in 1908 Minkowski suggested that a photon gains momentum when entering a medium; only a year later, in 1909, Abraham contended that, on the contrary, it actually loses momentum. Astonishingly, there are very good reasons to give credit to both viewpoints. Let us have a closer look at some of the most intuitive arguments in favor of each of the two theories.
If a photon traveling in vacuum enters a material, a slab of glass for example, its speed decreases by a factor, known as the refractive index. It follows rather logically that the photon’s momentum, which is proportional to its speed, should also decrease in the glass. There, one might justifiably think, Abraham must be right!
However, a photon is a quantum entity with a wavelength and a momentum given by the Planck’s constant divided by its wavelength. In the glass the wavelength decreases by a factor equal to the refractive index and, therefore, its momentum must increase. We would now seem to be justified in thinking that Minkowski must be right, after all.
Puzzling! Both arguments come across as sound and logical – yet they would also seem to lead to antithetical conclusions. Indeed, for over one hundred years, generations of great scientists racked their brains over this dilemma, unable to reach any sort of definitive resolution.
Since physics is an experimental science, it may be argued that it is for the experiments to decide on the issue. And there is, indeed, very good experimental evidence in favor of the theories – actually, in favor of both theories! Where, then, is the catch, if indeed there is one?
Some experimental evidence in favor of Minkowski comes from the cooling of atoms and from the optical stretcher. The latter, for example, is a device where a cell kept between two counter-propagating laser beams stretches along the axes of the beams. This appears to be consistent with the Minkowski momentum, since the stretching results from the decrease of the momentum when light crosses the boundary between the water and the cell. On the opposite side of the field, sympathetic to Abraham, we find a recent experiment that shows the recoiling of an optical fiber on the exit of a light pulse.
Barnett may well have found the answer. Surprisingly, both arguments are reasonable and sound because they are both right – but they refer to two different momenta, namely the kinetic momentum, where Abraham’s theory is concerned, and the canonical momentum, where Minkowski’s theory is concerned.
The kinetic momentum of a body is simply the product of its mass multiplied by its speed. The kinetic momentum of a photon when it enters a medium decreases in accordance with Abraham’s prediction, but such change is compensated by the recoiling of the medium, so that what is conserved is the total kinetic momentum of the photon and the medium.
The canonical momentum is a little subtler to grasp. It can be intimately related to the quantum nature of the photon. It appears in the interactions between single photons and single atoms, such as atom cooling. The canonical momentum of a photon increases when it interacts with an atom, and, as predicted by Minkowski once again what is conserved is the total canonical momentum of the photon and the atom.
"If light propagates in free space," explains Barnett, "there is no difference between the kinetic and the canonical momentum. However, such difference matters when light propagates inside a medium." This may go some way towards explaining why the fact that Abraham’s and Minkowski’s theories refer to two different momenta, went unnoticed before
Looking ahead, Barnett asks: "Might there be phenomena where both momenta show up at the same time?" Once the idea that both momenta are correct is accepted, it would make sense to plan experiments where both are present together. The focus on trying to prove/disprove Abraham or Minkowski may have prevented scientists from exploring such possibilities in the past – the good news is that this may be about to change soon.
Giovanni Volpe
2010 © Optics & Photonics Focus
GV is currently working as a postdoctoral researcher in optics, statistical physics and soft matter at the Max Planck Institute in Stuttgart (Germany).

Stephen M. Barnett, Resolution of the Abraham-Minkowski Dilemma, Physical Review Letters (2010) 104, 070401 (link).